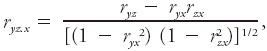Partial correlation: Difference between revisions
From Glossary of Meteorology
imported>Perlwikibot (Created page with " {{TermHeader}} {{TermSearch}} <div class="termentry"> <div class="term"> == partial correlation == </div> <div class="definition"><div class="short_definition">The [[c...") |
imported>Perlwikibot No edit summary |
||
| Line 9: | Line 9: | ||
</div> | </div> | ||
<div class="definition"><div class="short_definition">The [[correlation]] between the residuals of two [[random variables]] (variates) with respect to common regressors.</div><br/> <div class="paragraph">Denoting the [[regression function]] of two variates ''y'' and ''z'' with respect to a common set of regressors ''x''<sub>1</sub>, ''x''<sub>2</sub>, · · · ''x''<sub>''n''</sub> by ''Y'' and ''Z'', the coefficient of partial correlation between ''y'' and ''z'' is defined as the coefficient of simple [[linear correlation]] between (''y'' | <div class="definition"><div class="short_definition">The [[correlation]] between the residuals of two [[random variables]] (variates) with respect to common regressors.</div><br/> <div class="paragraph">Denoting the [[regression function]] of two variates ''y'' and ''z'' with respect to a common set of regressors ''x''<sub>1</sub>, ''x''<sub>2</sub>, · · · ''x''<sub>''n''</sub> by ''Y'' and ''Z'', the coefficient of partial correlation between ''y'' and ''z'' is defined as the coefficient of simple [[linear correlation]] between (''y'' - ''Y'') and (''z'' - ''Z''). To estimate the partial correlation, it is usually necessary to resort to [[sample]] approximations ''Y''′ and ''Z''′ of ''Y'' and ''Z''. In that case, the estimate of the partial correlation is the sample value of the coefficient of simple, linear correlation between (''y'' - ''Y''′) and (''z'' - ''Z''′). In the simplest case in which ''Y''′ and ''Z''′ are taken as [[linear]] functions of a single [[variable]] ''x'', the sample estimate ''r''<sub>''yz.x''</sub> of the partial correlation coefficient is given by the formula <div class="display-formula"><blockquote>[[File:ams2001glos-Pe4.gif|link=|center|ams2001glos-Pe4]]</blockquote></div> where the symbol ''r''<sub>''uv''</sub> denotes the sample coefficient of linear correlation between any pair of variates ''u'', ''v''. <br/>''See'' [[regression]].</div><br/> </div> | ||
</div> | </div> | ||
Latest revision as of 14:48, 20 February 2012
partial correlation
The correlation between the residuals of two random variables (variates) with respect to common regressors.
Denoting the regression function of two variates y and z with respect to a common set of regressors x1, x2, · · · xn by Y and Z, the coefficient of partial correlation between y and z is defined as the coefficient of simple linear correlation between (y - Y) and (z - Z). To estimate the partial correlation, it is usually necessary to resort to sample approximations Y′ and Z′ of Y and Z. In that case, the estimate of the partial correlation is the sample value of the coefficient of simple, linear correlation between (y - Y′) and (z - Z′). In the simplest case in which Y′ and Z′ are taken as linear functions of a single variable x, the sample estimate ryz.x of the partial correlation coefficient is given by the formula where the symbol ruv denotes the sample coefficient of linear correlation between any pair of variates u, v.
See regression.
See regression.