Froude number: Difference between revisions
From Glossary of Meteorology
imported>Liss45 |
imported>Liss45 |
||
| Line 11: | Line 11: | ||
#<div class="definition"><div class="short_definition">The nondimensional ratio of the [[inertial force]] to the [[force of gravity]] for a given fluid flow; the reciprocal of the [[Reech number]].</div><br/> <div class="paragraph">It may be given as <div class="display-formula"><blockquote>[[File:ams2001glos-Fe21.gif|link=|center|ams2001glos-Fe21]]</blockquote></div> where ''V'' is a [[characteristic velocity]], ''L'' a [[characteristic length]], and ''g'' the [[acceleration of gravity]]; or as the square root of this number.</div><br/> </div> | #<div class="definition"><div class="short_definition">The nondimensional ratio of the [[inertial force]] to the [[force of gravity]] for a given fluid flow; the reciprocal of the [[Reech number]].</div><br/> <div class="paragraph">It may be given as <div class="display-formula"><blockquote>[[File:ams2001glos-Fe21.gif|link=|center|ams2001glos-Fe21]]</blockquote></div> where ''V'' is a [[characteristic velocity]], ''L'' a [[characteristic length]], and ''g'' the [[acceleration of gravity]]; or as the square root of this number.</div><br/> </div> | ||
#<div class="definition"><div class="short_definition">For continuously stratified, nonrotating, dry, inviscid 2D flow over an obstacle of height ''h'', with incoming [[wind speed]] ''U'', and [[upstream]] [[Brunt-väisälä frequency]] ''N'', the quantity ''U''/(''Nh'') yields a measure of whether there will be an upstream-propagating region of decelerated flow and, hence, is also sometimes referred to as the Froude number. For ''U''/(''Nh'') >> 1, the flow ascends over the obstacle with no upstream deceleration. For ''U''/(''Nh'') << 1, a region of upstream flow deceleration forms that may propagate continuously upstream with time. Note that referring to the above relation as the Froude number is not consistent with the classical definition of Fr (see definition 1 above). For consistency with the original definition of Fr, some advocate referring to the relation ''Nh''/''U'' (the inverse of Fr as it is defined herein) as the nondimensional mountain height.</div><br/> | #<div class="definition"><div class="short_definition">For continuously stratified, nonrotating, dry, inviscid 2D flow over an obstacle of height ''h'', with incoming [[wind speed]] ''U'', and [[upstream]] [[Brunt-väisälä frequency]] ''N'', the quantity ''U''/(''Nh'') yields a measure of whether there will be an upstream-propagating region of decelerated flow and, hence, is also sometimes referred to as the Froude number. For ''U''/(''Nh'') >> 1, the flow ascends over the obstacle with no upstream deceleration. For ''U''/(''Nh'') << 1, a region of upstream flow deceleration forms that may propagate continuously upstream with time. Note that referring to the above relation as the Froude number is not consistent with the classical definition of Fr (see definition 1 above). For consistency with the original definition of Fr, some advocate referring to the relation ''Nh''/''U'' (the inverse of Fr as it is defined herein) as the nondimensional mountain height.</div><br/> | ||
</div | </div><br/>''term edited 12 Dec 2014'' | ||
{{TermIndex}} | {{TermIndex}} | ||
Latest revision as of 07:35, 12 December 2014
Froude number
- The nondimensional ratio of the inertial force to the force of gravity for a given fluid flow; the reciprocal of the Reech number.
It may be given aswhere V is a characteristic velocity, L a characteristic length, and g the acceleration of gravity; or as the square root of this number.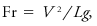
- For continuously stratified, nonrotating, dry, inviscid 2D flow over an obstacle of height h, with incoming wind speed U, and upstream Brunt-väisälä frequency N, the quantity U/(Nh) yields a measure of whether there will be an upstream-propagating region of decelerated flow and, hence, is also sometimes referred to as the Froude number. For U/(Nh) >> 1, the flow ascends over the obstacle with no upstream deceleration. For U/(Nh) << 1, a region of upstream flow deceleration forms that may propagate continuously upstream with time. Note that referring to the above relation as the Froude number is not consistent with the classical definition of Fr (see definition 1 above). For consistency with the original definition of Fr, some advocate referring to the relation Nh/U (the inverse of Fr as it is defined herein) as the nondimensional mountain height.
term edited 12 Dec 2014
