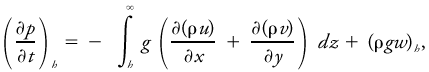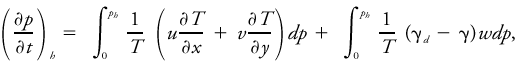Tendency equation: Difference between revisions
From Glossary of Meteorology
imported>Perlwikibot No edit summary |
imported>Perlwikibot No edit summary |
||
| Line 9: | Line 9: | ||
</div> | </div> | ||
<div class="definition"><div class="short_definition">An equation for the [[local change]] of [[pressure]] at any point in the [[atmosphere]], derived by combining the [[equation of continuity]] with an integrated form of the [[hydrostatic equation]].</div><br/> <div class="paragraph">In its basic form it is <div class="display-formula"><blockquote>[[File:ams2001glos-Te16.gif|link=|center|ams2001glos-Te16]]</blockquote></div> where (∂''p''/∂''t'')<sub>''h''</sub> represents the [[pressure tendency]] at a height ''h'', ''g'' is the [[acceleration of gravity]], ρ is the air density, and ''u'', ''v'', and ''w'' are, respectively, the ''x'', ''y'', and ''z'' components of the [[wind]] velocity. The subscript ''h'' indicates that the quantity is to be measured at the level ''z'' = ''h''. The first term on the right represents the local change of pressure due to the net horizontal [[mass convergence]] above the level ''h''. The second term represents the local change of pressure due to vertical motion through the level ''h''. In general, these two terms balance one another so that the pressure tendency is obtained as a small difference between two quantities of large magnitude, a very undesirable feature for computational purposes. This difficulty is partially eliminated by making use of a form of the tendency equation that assumes that individual [[density]] changes are [[adiabatic]], that ∂''p''/∂''z'' = ''dp''/''dz'', and that the [[advection]] of pressure by the wind is negligible. It may be written <div class="display-formula"><blockquote>[[File:ams2001glos-Te17.gif|link=|center|ams2001glos-Te17]]</blockquote></div> where ''T'' is the Kelvin [[temperature]]; γ<sub>d</sub> = -''dT''/''dz'' the [[dry-]] or [[saturation-adiabatic lapse rate]], depending on whether the air is unsaturated or saturated, respectively; and γ = -∂''T''/∂''z'', the [[environmental lapse rate]]. This equation is used most often to estimate the integrated vertical motion, given the pressure tendency and the advection.</div><br/> </div><div class="reference">Panofsky, H. 1956. Introduction to Dynamic Meteorology. 124–130. </div><br/> | <div class="definition"><div class="short_definition">An equation for the [[local change]] of [[pressure]] at any point in the [[atmosphere]], derived by combining the [[equation of continuity]] with an integrated form of the [[hydrostatic equation|hydrostatic equation]].</div><br/> <div class="paragraph">In its basic form it is <div class="display-formula"><blockquote>[[File:ams2001glos-Te16.gif|link=|center|ams2001glos-Te16]]</blockquote></div> where (∂''p''/∂''t'')<sub>''h''</sub> represents the [[pressure tendency]] at a height ''h'', ''g'' is the [[acceleration of gravity]], ρ is the air density, and ''u'', ''v'', and ''w'' are, respectively, the ''x'', ''y'', and ''z'' components of the [[wind]] velocity. The subscript ''h'' indicates that the quantity is to be measured at the level ''z'' = ''h''. The first term on the right represents the local change of pressure due to the net horizontal [[mass convergence]] above the level ''h''. The second term represents the local change of pressure due to vertical motion through the level ''h''. In general, these two terms balance one another so that the pressure tendency is obtained as a small difference between two quantities of large magnitude, a very undesirable feature for computational purposes. This difficulty is partially eliminated by making use of a form of the tendency equation that assumes that individual [[density]] changes are [[adiabatic]], that ∂''p''/∂''z'' = ''dp''/''dz'', and that the [[advection]] of pressure by the wind is negligible. It may be written <div class="display-formula"><blockquote>[[File:ams2001glos-Te17.gif|link=|center|ams2001glos-Te17]]</blockquote></div> where ''T'' is the Kelvin [[temperature]]; γ<sub>d</sub> = -''dT''/''dz'' the [[dry-adiabatic lapse rate|dry-]] or [[saturation-adiabatic lapse rate]], depending on whether the air is unsaturated or saturated, respectively; and γ = -∂''T''/∂''z'', the [[environmental lapse rate]]. This equation is used most often to estimate the integrated vertical motion, given the pressure tendency and the advection.</div><br/> </div><div class="reference">Panofsky, H. 1956. Introduction to Dynamic Meteorology. 124–130. </div><br/> | ||
</div> | </div> | ||
Latest revision as of 17:04, 25 April 2012
tendency equation[edit | edit source]
An equation for the local change of pressure at any point in the atmosphere, derived by combining the equation of continuity with an integrated form of the hydrostatic equation.
In its basic form it is where (∂p/∂t)h represents the pressure tendency at a height h, g is the acceleration of gravity, ρ is the air density, and u, v, and w are, respectively, the x, y, and z components of the wind velocity. The subscript h indicates that the quantity is to be measured at the level z = h. The first term on the right represents the local change of pressure due to the net horizontal mass convergence above the level h. The second term represents the local change of pressure due to vertical motion through the level h. In general, these two terms balance one another so that the pressure tendency is obtained as a small difference between two quantities of large magnitude, a very undesirable feature for computational purposes. This difficulty is partially eliminated by making use of a form of the tendency equation that assumes that individual density changes are adiabatic, that ∂p/∂z = dp/dz, and that the advection of pressure by the wind is negligible. It may be written where T is the Kelvin temperature; γd = -dT/dz the dry- or saturation-adiabatic lapse rate, depending on whether the air is unsaturated or saturated, respectively; and γ = -∂T/∂z, the environmental lapse rate. This equation is used most often to estimate the integrated vertical motion, given the pressure tendency and the advection.
Panofsky, H. 1956. Introduction to Dynamic Meteorology. 124–130.