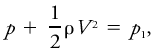Static pressure: Difference between revisions
From Glossary of Meteorology
imported>Perlwikibot (Created page with " {{TermHeader}} {{TermSearch}} <div class="termentry"> <div class="term"> == static pressure == </div> <div class="definition"><div class="short_definition">In engineer...") |
imported>Perlwikibot No edit summary |
||
| (One intermediate revision by the same user not shown) | |||
| Line 9: | Line 9: | ||
</div> | </div> | ||
<div class="definition"><div class="short_definition">In engineering fluid mechanics, the [[pressure]] in a homogeneous [[incompressible fluid]] in [[steady flow]] along a level [[streamline]] at points other than the stagnation point.</div><br/> <div class="paragraph">Thus if ''p'' is the static pressure, [[Bernoulli's equation]] gives <div class="display-formula"><blockquote>[[File:ams2001glos-Se61.gif|link=|center|ams2001glos-Se61]]</blockquote></div> where ρ is the [[density]] of the fluid, ''V'' the speed, and ''p''<sub>1</sub> the pressure at the stagnation point, called the [[total pressure]]. The [[kinetic energy]] per unit volume (1/2)ρ''V''<sup>2</sup> is <br/>''also called'' the [[dynamic pressure]]. The static pressure is that measured by a [[barometer]] moving with the fluid. Since the static pressure is the pressure in the moving fluid and is distributed along the streamline exactly as the [[hydrodynamic pressure]], the terminology is most unfortunately chosen. Since it is rigorously defined only when Bernoulli's equation applies, meteorologists do well in avoiding the term. The unqualified term | <div class="definition"><div class="short_definition">In engineering fluid mechanics, the [[pressure]] in a homogeneous [[incompressible fluid|incompressible fluid]] in [[steady flow]] along a level [[streamline]] at points other than the stagnation point.</div><br/> <div class="paragraph">Thus if ''p'' is the static pressure, [[Bernoulli's equation]] gives <div class="display-formula"><blockquote>[[File:ams2001glos-Se61.gif|link=|center|ams2001glos-Se61]]</blockquote></div> where ρ is the [[density]] of the fluid, ''V'' the speed, and ''p''<sub>1</sub> the pressure at the stagnation point, called the [[total pressure]]. The [[kinetic energy]] per unit volume (1/2)ρ''V''<sup>2</sup> is <br/>''also called'' the [[dynamic pressure|dynamic pressure]]. The static pressure is that measured by a [[barometer]] moving with the fluid. Since the static pressure is the pressure in the moving fluid and is distributed along the streamline exactly as the [[hydrodynamic pressure]], the terminology is most unfortunately chosen. Since it is rigorously defined only when Bernoulli's equation applies, meteorologists do well in avoiding the term. The unqualified term "pressure" is quite satisfactory in this connection. However, the instrumental precautions taken in measuring the static pressure in fluid mechanics must also be applied to meteorological barometers so that it is the pressure and not the [[wind speed]] that is being measured. The measured meteorological pressure is in approximate [[hydrostatic equilibrium]] because of the relatively small vertical accelerations in the [[atmosphere]], but this condition does not ordinarily obtain in those studies in which the concept of static pressure is used. Thus static pressure and [[hydrostatic pressure]] must be distinguished.</div><br/> </div> | ||
</div> | </div> | ||
Latest revision as of 16:58, 25 April 2012
static pressure
In engineering fluid mechanics, the pressure in a homogeneous incompressible fluid in steady flow along a level streamline at points other than the stagnation point.
Thus if p is the static pressure, Bernoulli's equation gives where ρ is the density of the fluid, V the speed, and p1 the pressure at the stagnation point, called the total pressure. The kinetic energy per unit volume (1/2)ρV2 is
also called the dynamic pressure. The static pressure is that measured by a barometer moving with the fluid. Since the static pressure is the pressure in the moving fluid and is distributed along the streamline exactly as the hydrodynamic pressure, the terminology is most unfortunately chosen. Since it is rigorously defined only when Bernoulli's equation applies, meteorologists do well in avoiding the term. The unqualified term "pressure" is quite satisfactory in this connection. However, the instrumental precautions taken in measuring the static pressure in fluid mechanics must also be applied to meteorological barometers so that it is the pressure and not the wind speed that is being measured. The measured meteorological pressure is in approximate hydrostatic equilibrium because of the relatively small vertical accelerations in the atmosphere, but this condition does not ordinarily obtain in those studies in which the concept of static pressure is used. Thus static pressure and hydrostatic pressure must be distinguished.
also called the dynamic pressure. The static pressure is that measured by a barometer moving with the fluid. Since the static pressure is the pressure in the moving fluid and is distributed along the streamline exactly as the hydrodynamic pressure, the terminology is most unfortunately chosen. Since it is rigorously defined only when Bernoulli's equation applies, meteorologists do well in avoiding the term. The unqualified term "pressure" is quite satisfactory in this connection. However, the instrumental precautions taken in measuring the static pressure in fluid mechanics must also be applied to meteorological barometers so that it is the pressure and not the wind speed that is being measured. The measured meteorological pressure is in approximate hydrostatic equilibrium because of the relatively small vertical accelerations in the atmosphere, but this condition does not ordinarily obtain in those studies in which the concept of static pressure is used. Thus static pressure and hydrostatic pressure must be distinguished.