Often the greatest magnitude at a given point of any spatially and temporally varying physical quantity governed by a wave equation; can also mean the spatial part of a time-harmonic wave function.
For example, in the time-harmonic (or sinusoidal) scalar wave function with circular frequency ω,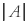
, is also called the amplitude of the wave. In its most general sense, amplitude means extent or size. Thus the amplitude of a wave is some measure of its size.
where φ(x) is the (complex) amplitude of the wave, although the modulus of φ also may be called its amplitude. The (complex) amplitude of the scalar plane harmonic wave
with wavenumber k and initial phase θ is A exp(ikx - iθ), the modulus of which,
, is also called the amplitude of the wave. In its most general sense, amplitude means extent or size. Thus the amplitude of a wave is some measure of its size.
