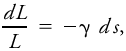Rain attenuation: Difference between revisions
From Glossary of Meteorology
imported>Perlwikibot (Created page with " {{TermHeader}} {{TermSearch}} <div class="termentry"> <div class="term"> == rain attenuation == </div> <div class="definition"><div class="short_definition">The deplet...") |
imported>Perlwikibot No edit summary |
||
| Line 9: | Line 9: | ||
</div> | </div> | ||
<div class="definition"><div class="short_definition">The depletion of [[electromagnetic energy]] during propagation through [[rain]], caused by [[raindrop]] scattering and [[absorption]].</div><br/> <div class="paragraph">The effect is described by [[Beer's law]] as the fractional depletion of [[radiance]] per unit pathlength, <div class="display-formula"><blockquote>[[File:ams2001glos-Re12.gif|link=|center|ams2001glos-Re12]]</blockquote></div> where ''L'' is the [[monochromatic]] radiance at a given [[wavelength]], γ is the volume [[attenuation coefficient]] (or [[extinction coefficient]]) due to [[absorption]] and [[scattering]] by [[rain]], and ''ds'' is an increment of pathlength. In [[radar]], the depletion of [[power]] from incident plane-wave [[radiation]] is sometimes described by the [[specific attenuation]] ''Y'', usually expressed in units of decibels per kilometer, related to the volume attenuation coefficient by <div class="display-formula"><blockquote>[[File:ams2001glos-Re13.gif|link=|center|ams2001glos-Re13]]</blockquote></div> where ''Y'' is in decibels per kilometer when γ is in inverse kilometers. The proportionality factor is 10 log<sub>10</sub>''e''. The specific attenuation for a given radar wavelength depends on the [[temperature]] and the [[drop-size distribution]]. If this distribution is known or can be approximated as a function of [[rainfall rate]], the specific attenuation can be estimated as a function of rainfall rate.</div><br/> </div> | <div class="definition"><div class="short_definition">The depletion of [[electromagnetic energy]] during propagation through [[rain]], caused by [[raindrop]] scattering and [[absorption]].</div><br/> <div class="paragraph">The effect is described by [[Beer's law]] as the fractional depletion of [[radiance]] per unit pathlength, <div class="display-formula"><blockquote>[[File:ams2001glos-Re12.gif|link=|center|ams2001glos-Re12]]</blockquote></div> where ''L'' is the [[monochromatic]] radiance at a given [[wavelength]], γ is the volume [[attenuation coefficient|attenuation coefficient]] (or [[extinction coefficient]]) due to [[absorption]] and [[scattering]] by [[rain]], and ''ds'' is an increment of pathlength. In [[radar]], the depletion of [[power]] from incident plane-wave [[radiation]] is sometimes described by the [[specific attenuation]] ''Y'', usually expressed in units of decibels per kilometer, related to the volume attenuation coefficient by <div class="display-formula"><blockquote>[[File:ams2001glos-Re13.gif|link=|center|ams2001glos-Re13]]</blockquote></div> where ''Y'' is in decibels per kilometer when γ is in inverse kilometers. The proportionality factor is 10 log<sub>10</sub>''e''. The specific attenuation for a given radar wavelength depends on the [[temperature]] and the [[drop-size distribution]]. If this distribution is known or can be approximated as a function of [[rainfall rate]], the specific attenuation can be estimated as a function of rainfall rate.</div><br/> </div> | ||
</div> | </div> | ||
Latest revision as of 16:44, 25 April 2012
rain attenuation[edit | edit source]
The depletion of electromagnetic energy during propagation through rain, caused by raindrop scattering and absorption.
The effect is described by Beer's law as the fractional depletion of radiance per unit pathlength, where L is the monochromatic radiance at a given wavelength, γ is the volume attenuation coefficient (or extinction coefficient) due to absorption and scattering by rain, and ds is an increment of pathlength. In radar, the depletion of power from incident plane-wave radiation is sometimes described by the specific attenuation Y, usually expressed in units of decibels per kilometer, related to the volume attenuation coefficient by where Y is in decibels per kilometer when γ is in inverse kilometers. The proportionality factor is 10 log10e. The specific attenuation for a given radar wavelength depends on the temperature and the drop-size distribution. If this distribution is known or can be approximated as a function of rainfall rate, the specific attenuation can be estimated as a function of rainfall rate.