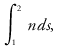Fermat's principle

In its more or less original form, light travels between two points in the least possible time.

More correctly, the path taken by a light ray between two points in an optically homogeneous media is such that the line integral along the path,

where n is the refractive index, is an extremum (a minimum or a maximum or even stationary) relative to all possible paths. Because n is inversely related to phase velocity, this integral is proportional to a time, the constant of proportionality being the free-space speed of light. Fermat was guided by a "metaphysical principle that nature performed its actions in the simplest and most economical ways."

Sabra, A. I. 1967. Theories of Light: From Descartes to Newton. p. 136.

INDEX
A
B
C
D
E
F
G
H
I
J
K
L
M
N
O
P
Q
R
S
T
U
V
W
X
Y
Z
0
1
2
3
4
5
6
7
8
9