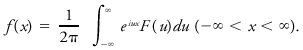Fourier transform
From Glossary of Meteorology
Fourier transform
An analytical transformation of a function f(x) obtained (if it exists) by multiplying the function by e-iux and integrating over all x, where u is the new variable of the transform F(u) and i2 = -1.
If the Fourier transform of a function is known, the function itself may be recovered by use of the inversion formula: The Fourier transform has the same uses as the Fourier series: For example, the integrand F(u) exp (iux) is a solution of a given linear equation, so that the integral sum of these solutions is the most general solution of the equation. When the variable u is complex, the Fourier transform is equivalent to the Laplace transform.
See also Fourier integral, spectral function.
See also Fourier integral, spectral function.