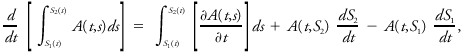Leibniz's theorem of calculus
From Glossary of Meteorology
Leibniz's theorem of calculus
A relationship between the derivative of an integral and the integral of a derivative, that is, where S1 and S2 are limits of integration, s is a dummy distance or space variable such as height z, t is time, and A is some meteorological quantity, such as potential temperature or humidity, that is a function of both space and time. If the limits of integration are constant with time, then the last two terms are zero, and the derivative of the integral equals the integral of the derivative. However, there are many atmospheric situations, such as a growing atmospheric boundary layer, where the limits of integration can change with time. Namely, if one wishes to integrate over the depth of the boundary layer (between limits z = 0 to z = zi) to find a boundary layer average, for example, but the top of the boundary layer at height zi(t) is rising with time, then one must use the full form of Leibniz's theorem to account for this effect.