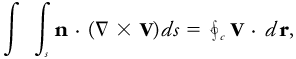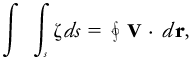Stokes's theorem

The statement that if s is a surface in three dimensions having a closed curve c as its boundary, then the circulation of a vector V around c is equal to the flux of the vorticity (curl of V) through s, under certain mathematical conditions on these fields and boundaries:

where n is the unit vector normal to s on that side of s arbitrarily taken as the positive side. The positive direction along c is defined as the direction along which an observer, traveling on the positive side of s, would proceed while keeping the enclosed area to his left. dr is a vector line element of c.

For two-dimensional flow in the x, y plane, Stokes's theorem becomes

where ζ is the vertical component of the vorticity. This states that the circulation around a given curve per unit area enclosed by the curve is equal to the average vorticity within the area.

INDEX
A
B
C
D
E
F
G
H
I
J
K
L
M
N
O
P
Q
R
S
T
U
V
W
X
Y
Z
0
1
2
3
4
5
6
7
8
9