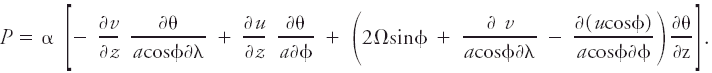potential vorticity
(Sometimes called absolute potential vorticity.) The specific volume times the scalar product of the absolute vorticity vector and the gradient of potential temperature: where α is the specific volume, Ω the angular velocity vector of the earth's rotation, u the three- dimensional vector velocity relative to the rotating earth, and θ the potential temperature.
In the absence of friction and heat sources, the Ertel potential vorticity P is a materially conservative property (it remains constant for each particle). In spherical coordinates (λ, φ, r), where λ is longitude, φ is latitude, and r is the distance from the center of the earth, the above expression for P becomes This nonhydrostatic version is not necessary for the analysis of large-scale weather systems, and an approximate hydrostatic version is usually used. This approximate version neglects terms involving the vertical velocity w, neglects the Coriolis terms proportional to the cosine of the latitude, and makes selective use of r ≈ a, where a is the constant radius of the earth. In this way we obtain the approximate form The potential vorticity has the SI units m2 s-1K kg-1. It has become accepted to define 1.0 × 10-6 m2 s-1K kg-1 as one potential vorticity unit (1 PVU).
See vorticity equation.
See vorticity equation.
Gill, A. E. 1982. Atmosphere–Ocean Dynamics. Academic Press, . 237–241.