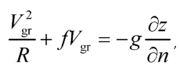Gradient wind
From Glossary of Meteorology
gradient wind
Any horizontal wind velocity tangent to the contour line of a constant-pressure surface (or to the isobar of a geopotential surface) at the point in question.
At such points where the wind is gradient, the Coriolis acceleration and the centripetal acceleration together exactly balance the horizontal pressure force: where Vgr is the gradient wind speed, R is the radius of curvature of the path, f is the Coriolis parameter, g is the acceleration of gravity, z is the elevation of the constant-pressure surface, and n is the direction normal to the streamline and contour toward decreasing elevation, that is, to the left of the direction of flow in the Northern Hemisphere and to the right in the Southern Hemisphere. Since R > 0 for cyclonic flow and R < 0 for anticyclonic flow, it follows that the cyclonic gradient speed is less than (and the anticyclonic gradient speed is greater than) the geostrophic wind speed for the same latitude and pressure force.
Holton, J. R. 1992. An Introduction to Dynamic Meteorology. 3d edition, Academic Press, . 67–69.
(Term edited 5/23/2014)